[Plugin] TrueTangents v3.0
-
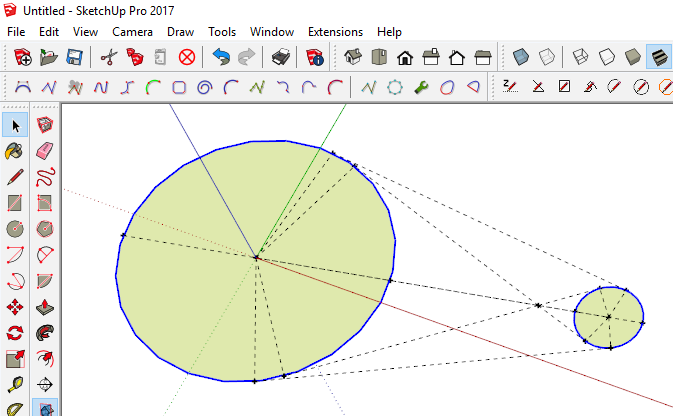
The Tan-Tan-Radius problem is a simple circle-circle intersection problem as you may know if you have ever done this manually. If not, simply offset the two arcs/circles by the radius of the circle to be placed tangent to the two. Where those offsets intersect is the center of the tangent circle.
-
Hi TIG-
TT 3.0's "filletarc" is exactly the tool I need, but it has a 'feature' that doesn't make sense to me: It appears that at least for circles in the <1" diameter range the minimum fillet radius is limited to 1/2 the circle's radius.
In other words the smallest fillet I can add to a 1" circle is 1/4".
On the other hand I can use TT's "tangentialarcs" tool to make a 1/16" fillet -- but I have to take the added step of constructing a 1/16" inch long line segment on my fillet line and select that in order to force "tangentialarcs" to construct a fillet of the correct radius -- except that this actually creates an approximately correct radius, whereas Filletarc's radius is exactly what I input.
Anyway, I guess my point is that I don't understand why Filletarc shouldn't be able to make fillets whose radii are smaller that 1/2 the filleted circle's radius.
Regardless, it's a really useful tool for dealing with the true geometry of circles!
Thanks, -Carl-
-
Looks great, although I'm not really sure whats this plugin good for? (Hey, I ain't bi engineer
 )
) -
@gonashvili said:
Looks great, although I'm not really sure whats this plugin good for? (Hey, I ain't bi engineer
 )
)Seriously?

-
@gonashvili said:
Looks great, although I'm not really sure whats this plugin good for? (Hey, I ain't bi engineer
 )
)Well there are 66 lines of explanations and more than 10 images in the first TIG's post, plus 5 pages of thread so...seriously?

-
If you really need these tools then you'll already understand what they do... otherwise don't bother installing the toolset...
-
@tig said:
If you really need these tools then you'll already understand what they do... otherwise don't bother installing the toolset...
ha!.. a decent way to put it

(sorry about my missing imgs in the thread.. I used to upload and display via mobile me then apple just wiped the whole thing out... meh.. I upload directly to scf nowadays)
-
Thanks TIG! I don't think I've ever seen such thorough explanations of plugin functionality. Much appreciated.
-
I believe that make that


-
Hi TIG. I installed TrueTangents via latest SketchUcation Plugin Store. It works, but why are the tool-tips displayed in the Ruby console and not in the status bar?
Latest SU Pro, Win 7 64 bit latest.
Thanks.
-
@dedmin said:
Hi TIG. I installed TrueTangents via latest SketchUcation Plugin Store. It works, but why are the tool-tips displayed in the Ruby console and not in the status bar?
Latest SU Pro, Win 7 64 bit latest.
Thanks.[attachment=0:ahmfglq7]<!-- ia0 -->Capture-2.jpg<!-- ia0 -->[/attachment:ahmfglq7]
This toolset was originally designed to run from the Ruby Console, or from within other methods - so the tools' guidance/errors go into the Ruby Console, rather than the status-bar or an error message-box...
It's the same in all SketchUp versions - it's not v2014 specific.

-
@tig said:
@dedmin said:
Hi TIG. I installed TrueTangents via latest SketchUcation Plugin Store. It works, but why are the tool-tips displayed in the Ruby console and not in the status bar?
Latest SU Pro, Win 7 64 bit latest.
Thanks.[attachment=0:2c4kpk1c]<!-- ia0 -->Capture-2.jpg<!-- ia0 -->[/attachment:2c4kpk1c]
This toolset was originally designed to run from the Ruby Console, or from within other methods - so the tools' guidance/errors go into the Ruby Console, rather than the status-bar or an error message-box...
It's the same in all SketchUp versions - it's not v2014 specific.

I see.

-
Does the True Tangent plugin work in SU2017?
Bob
-
-
Is it possible to draw an arc tangent to two others?
Thank you very much -
Do you mean a common-tangent to three arcs ?
It will be possible in some 'special' cases, but then you can contrive three arcs which can never all share a common-tangent...
Please give a clear example...
Or explain your question more clearly... -
I mean to draw an arc tangent to two circles.
Like in the image.
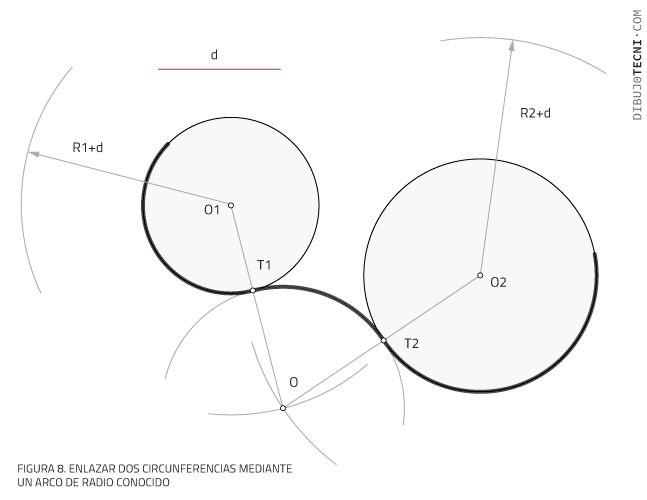
-
In the previous drawing the circles O1 and O2 are known
-
Sorry, very grateful for your attention!
-
It would also be interesting if it allows solving situations like this:

Advertisement