3D Truss Models
-
If we consider the peak joint as rigid (web joints are pin jointed), one can see that the moment at the peak is fairly substantial (145 ft-lbs):
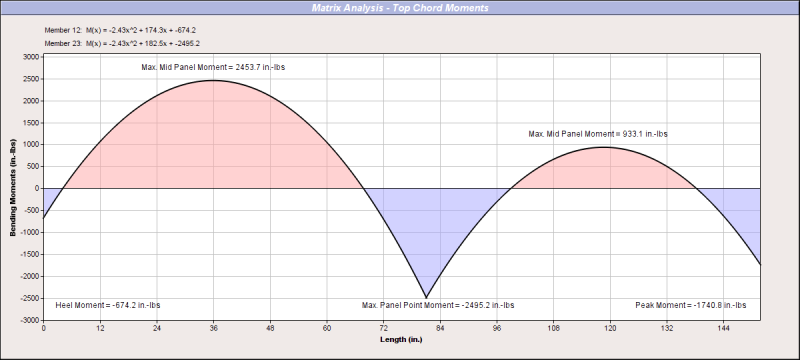
Both the top chords are in compression and pushing against each other, so the member on member contact is transferring a significant amount of that compressive axial load.
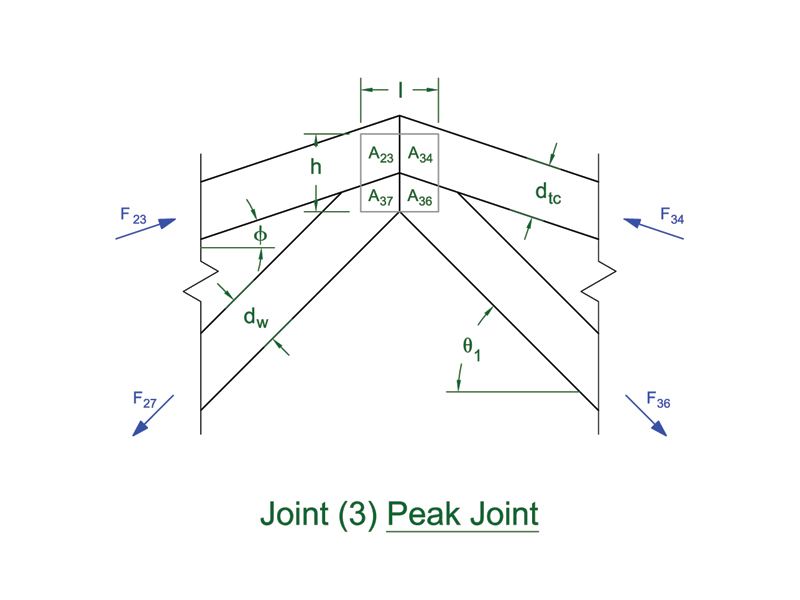
To account for the component of the load that is not being transferred at this joint I can probably use the equation from the TPI 1 for MPC trusses:
Pc' = sqrt(P[sub:y7ifrpex]P[/sub:y7ifrpex][sup:y7ifrpex]2[/sup:y7ifrpex] + (P[sub:y7ifrpex]N[/sub:y7ifrpex] x C[sub:y7ifrpex]r[/sub:y7ifrpex])[sup:y7ifrpex]2[/sup:y7ifrpex] (Sec. 8.3.3.3)
where:
P[sub:y7ifrpex]c[/sub:y7ifrpex]' = Resultant compressive force used for determination of minimum required metal plate contact area (lbs)
C[sub:y7ifrpex]R[/sub:y7ifrpex] = Reduction factor for cmopression force component across the joint interface for metal connector plate design: 0≤CR≤1.0 (lbs)
P[sub:y7ifrpex]iN[/sub:y7ifrpex] = Compression force component of the wood member under investigation normal to the wood member interface (lbs)
P[sub:y7ifrpex]iP[/sub:y7ifrpex] = Compression force component of the wood member under investigation parallel to the wood member interface (lbs)With the resultant being:
CR = 0.5
Pi = F23 = 2021.83 lbs.
PiN = Picos(Φ) = 2021.8 x cos(18.43) = 1918.1 lbs.
PiP = Pisin(Φ) = 2021.8 x sin(18.43) = 639.4 lbs.θ = atan(PiP/PiN x CR) - Φ = atan(639.4/1918.1 x 0.5) - 18.43 = 15.3 deg. (angle between resultant compressive force and chord member)
Pc' = √(PiP)2 + (PiN x CR)2 = √(639.4)2 + (1918.1 x 0.5)2 = 1152.6 lbs. @ 15.3 deg.
I kind of get the logic behind this equation but I question where does the Cr (reduction factor) come from? and why a value of 0.5?
I'm also looking at the rather large moment at this joint between the two top chords and wondering how best to factor that in.
-
To allow for these same moment effects at the heel of a truss the TPI stipulates a heel reduction factor H[sub:2y81ofvw]R[/sub:2y81ofvw] = 0.85 - 0.05(12tanθ -2.0) Sec. 8.3.2.2. I'm going to assume this is an empirical formula developed over years of testing and experience. However, for all non-heel joints this reduction factor is 1.0, which makes sense for an MPC truss but maybe not for a WGC truss, where moments at the joints are going to be larger due to the rigidity of the connection.
In the TPI 1-2014 commentary there is some good discussion on combined axial and moment loading scenarios. Equation E C8.7-1 approaches this problem by converting the moment into an equivalent tension force: Teq = 6M/d.
The logic behind this is to start with the bending stress and multiply it with the cross sectional area of the plates: (1) T[sub:2y81ofvw]eq[/sub:2y81ofvw] = f[sub:2y81ofvw]b[/sub:2y81ofvw] x 2dt
Where: f[sub:2y81ofvw]b[/sub:2y81ofvw] = M/s and S = 2(td[sup:2y81ofvw]2[/sup:2y81ofvw]/6)
Substituting into equation (1) yields the 6M/d expression.
The commentary notes that this method has proven to be conservative (which I like) in most situations however they have since supplanted it with a more complicated and accurate approach.
For analysis of the tensile capacity of the actual gusset plates (plywood or OSB), tension check, this addition of an equivalent tensile force seems to make sense to me.
T[sub:2y81ofvw]tot[/sub:2y81ofvw] = T[sub:2y81ofvw]axial[/sub:2y81ofvw] + T[sub:2y81ofvw]eq[/sub:2y81ofvw]
This is essentially the same as equation 3.9-1 in the NDS and can be written in this form:
The applied stress would then be: T/2td + 6M/2td[sup:2y81ofvw]2[/sup:2y81ofvw] which must be less than Ft' the allowable tensile stress of the plywood.
With the analysis of the fasteners (nails) one could probably use a simplified approach like this or a more complicated nail group analysis like I've used in calculating portal frames.
-
With the nail group calculation knowing the number of required nails, row spacing, nail spacing in a row one can come up with a rectangular approximation of the area filled with this group of nails.
The force applied to the worst case nail would:
F[sub:10dybyec]nail[/sub:10dybyec] = M[sub:10dybyec]joint[/sub:10dybyec]S[sub:10dybyec]x[/sub:10dybyec]S[sub:10dybyec]y[/sub:10dybyec]r[sub:10dybyec]max[/sub:10dybyec]/J
Where the Polar Moment of the Nail Group is given by J = bh[sup:10dybyec]3[/sup:10dybyec]/12 + b[sup:10dybyec]3[/sup:10dybyec]h/12: b = r[sub:10dybyec]x[/sub:10dybyec] + s[sub:10dybyec]x[/sub:10dybyec], h = r[sub:10dybyec]y[/sub:10dybyec] + s[sub:10dybyec]y[/sub:10dybyec]
and
s[sub:10dybyec]x[/sub:10dybyec] = nail spacing in x-dir
s[sub:10dybyec]y[/sub:10dybyec] = nail spacing in y-dir
r[sub:10dybyec]max[/sub:10dybyec] = distance from centroid of rectangle to corner (furthest fastener)This won't be entirely accurate since the fasteners will probably not form a perfect rectangle but it should be reasonably close.
Then one can add this worst case nail force to the average force on the nails from an axial member load (conservative) and come up with the combined load on the worst case fastener.
This becomes an iterative process:
Step 1: Compute the number of fasteners for only the tensile load.
Step 2: Given the number of fasteners and fastener group geometry from Step 1 then calculate the load on the worst case fastener in the group.
Step 3: Add the value in Step 2 to average value from Step 1 and check against the allowable for the fastener. If it exceeds the allowable then add a fastener and repeat the computation of the average fastener load due to tensile load only and the load due to the moments, repeat until it passes.It's no wonder that the truss companies have all of this stuff programmed. Technically this needs to be done for each joint at each load case, since load reversals may turn a compression loaded joint into a tension joint and vice versa, and the moments will be different for each load case.
As you can see engineering a truss (WGC or MPC) is no trivial matter.
-
I next want to turn my attention to mid-panel splices:
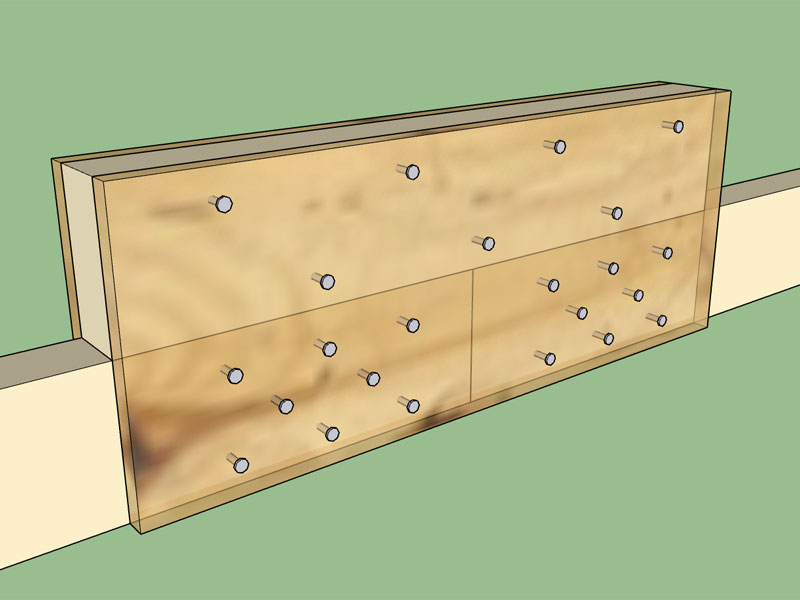
Nothing to exciting going on with this bottom chord splice, the splice will be loaded in tension with a moment, the same methods developed previously would apply.
In the case that the OSB or Plywood doesn't have enough strength for the combined axial + moment load then a vertical extension like what is shown could be improvised. This will not only increase the moment capacity of the gusset plate(s) but also the tensile strength. Note, that the number of fasteners into each bottom chord is not modified or decreased by the vertical extension of the gusset plate.
To be conservative I would not consider the 2x4 insert in the calculations of the updated tensile or moment strength of the gusset plate(s) even though it will contribute some unspecified amount. The filler block is primarily to secure the protruding gusset plates and to prevent them from buckling out-of-plane. To that end I don't feel that specifying the number of fasteners is overly critical. A reasonable amount of fasteners and some glue will create a cohesive unit.
In the case of top chord splice with the members being in compression the previous equation would dictate using a force that is 1/2 the compressive force, this would seem reasonably conservative but other load cases that could potentially load the splice in tension should also be investigated and compared. Again, any moments at the splice would also factor into the gusset plate sizing and fastener quantities.
-
The member calcs for a WGC truss will be very similar to an mpc truss except that the matrix analysis model will have rigid joints instead of pin joints. This will introduce some minor bending into the internal webs but nothing significant since these member are not being loaded along their spans. The deflection of the truss should probably improve slightly with its higher rigidity.
-
Spent some time Sunday night and yesterday evening working on the stepdown hip truss set. Given the shear number of trusses involved in one of the these assemblies I'm figuring it will be the rest of the week before I will see the light at the end of the tunnel, but progress is steady.
-
The stepdown hip truss set is mostly finished. I probably have a couple more days of work setting up raised heels, internal web algorithms, and advanced options.
-
Advanced options are now configured: fascia, sheathing, cladding, gypsum, ceiling battens.
Girder trusses can be single or double ply, I can add more plies if requested.
I've also added the option to use a drop-in purlin frame, see image and model below:
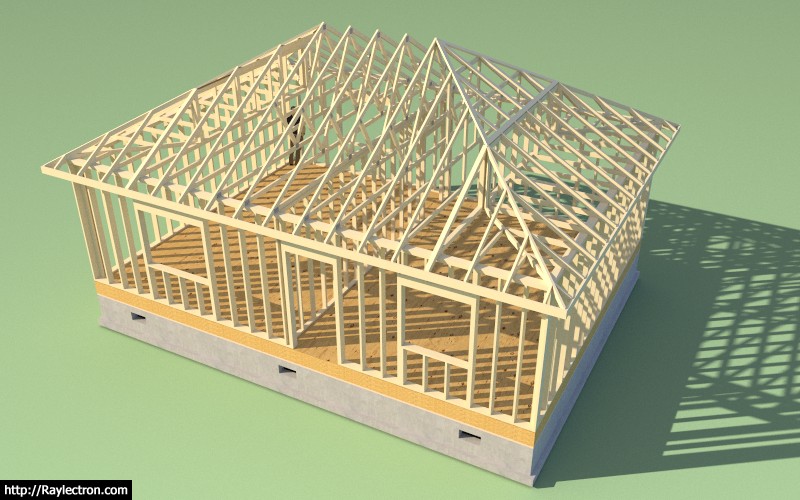
View model here:
3D Warehouse
3D Warehouse is a website of searchable, pre-made 3D models that works seamlessly with SketchUp.
(3dwarehouse.sketchup.com)
Items still needing attention:
- Error check in metric mode.
- Internal webs for hip trusses
- Internal web algorithm for end and hip jack trusses.
- Raised heels
- Enable soffit cut for jack trusses and hip trusses.
- Enable plates for hip, jack and drop-in purlin.
- Setup the half-a-hip option (one end is a gable end).
-
The complexity of the hip truss sets makes them a bit of pain to program but when its all said and done it is a thing of beauty.
Once I have the stepdown hip set well on its way it will not be to difficult to enable the other styles of truss hip sets. The only one that is significantly different is the terminal hip set and the dutch hip set but even those will fall into place relatively easily.
Once I get all of the hip sets configured I'm half tempted to bump my price another $10, would that be too much of a stretch? I'm already at $30 for the plugin and I would like to keep it within reach of even the casual user.
-
Version 1.9.2 - 06.24.2017
- Added Stepdown hip set trusses.
- Enabled advanced options for stepdown hip sets.
-
Using the stepdown truss set as a primitive it is possible to generate some fairly complex roofs:
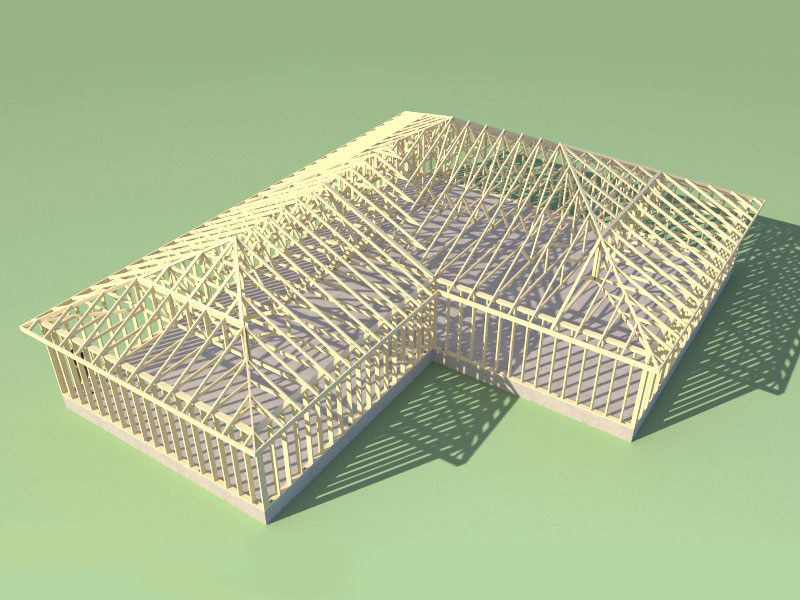
In this case I created two hip sets and then tied them together with a valley set. View model here:
3D Warehouse
3D Warehouse is a website of searchable, pre-made 3D models that works seamlessly with SketchUp.
(3dwarehouse.sketchup.com)
This one way to do it but the more typical way would be to further customize the larger hip set so as to eliminate the valley set entirely.
Interesting how the larger girder truss ties into the smaller girder truss (3 ply). The smaller girder truss would need to be beefed up to handle this point load if there is no bearing wall below, and then the point loads from the posts supporting the girder truss (in wall) would probably need spread footings.
A few seconds to create the basic geometry and then about 10 minutes of trimming (with the trim function) and manual adjustments. I can see that the truncated hip set feature would be nice to have where hip roofs are tying into other roofs, similar to what I have enabled for the gable truss tool.
-
In my neck of the woods we don't use valley sets - where one roof covers the main truss set it is usually stick-framed (usually 2x4's and 2x6's) with what we call 'California Fill' that bears on the main set. PM me if you'd like to see a typical detail.
-
I've seen them done this way up here in Washington but probably not as often.
I haven't shown the vertical studs and their pads only the sleepers, rafters and ridgeboard are shown, view model here:
3D Warehouse
3D Warehouse is a website of searchable, pre-made 3D models that works seamlessly with SketchUp.
(3dwarehouse.sketchup.com)
Where the Fill connects back to the other roof do I have that correct? Do you install another rafter against the truss?
Notice that I did not bevel the 2x12 sleeper, just shifted it so that if lines up correctly with the roof plane, of course if this is mounted on top of the sheathing then the position will change accordingly.
Those double bevel cuts on the rafters would scare me, but then again I'm no carpenter.
Funny how everything has a "California" in front of it:
- California Corner (Ext. Wall Corner) http://design.medeek.com/resources/framing/FRAMING_CORNERS.pdf
- California Fill
- California Hip Set https://3dwarehouse.sketchup.com/model/97644895-c988-435c-bdc4-c7b8956a6d46/California-Hip-Set-Rev-2
I guess the Californians are innovators.
-
That's pretty much how it's done down here, and with the detail I sent you it sounds like what you've seen and what we do are pretty close. I would agree, too, that the 2x sleepers rarely have the edge beveled to be in-plane - it takes too much time for the framers to figure the correct cut.
-
I was working on the hip sets last summer before my neck injury took me out so I'm glad to finally get back to it and at least get one variant knocked out.
I probably should have gotten on to it in January but I was still not feeling 100% so I been only tackling the easy stuff and chipping at those one by one.
I still have all the other variants to complete as well as raised heels, then the plates.
Timber trusses with bolted connector plates is on my hot list as is completion of the attic and gambrel attic trusses.
My full todo list for the plugin is at least two pages of hand written notes so there is plenty more to do.
The holy grail would be to have the plugin convert any complex roof outline (face) into a full truss or rafter roof without any manual editing, that is my target.
-
Added energy/raised heels for stepdown hip set (3 variants: wedge, slider and vertical w/ strut).
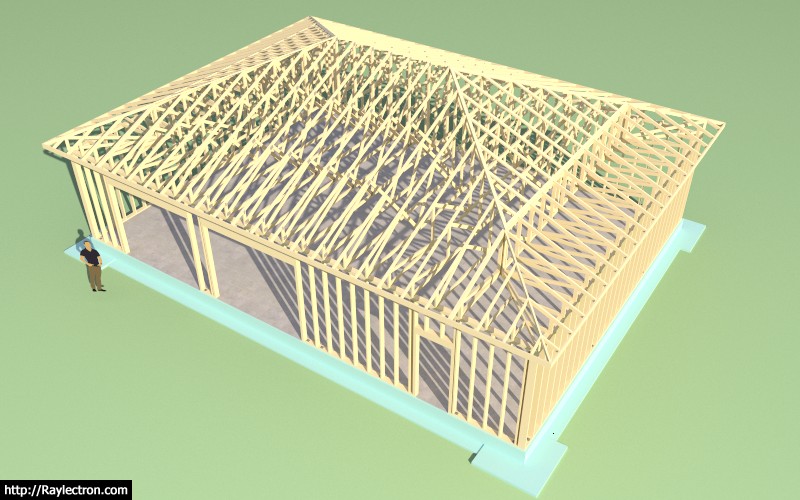
View model here:
3D Warehouse
3D Warehouse is a website of searchable, pre-made 3D models that works seamlessly with SketchUp.
(3dwarehouse.sketchup.com)
Now the possibilities are limitless since you can match the heel height of any other roof (ie. rafter, TJI etc...)
Adding in this feature was not as bad I thought it would be but each truss type in the set must be considered and dealt with separately:
- Common Trusses
- Hip Trusses
- End Jacks
- Hip Jacks
- Corner Jacks
-
I am wondering if any one has some shop drawings for a truss roof like the one below:
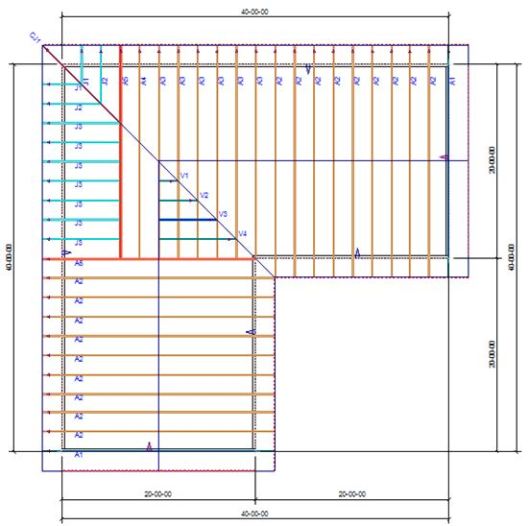
I am trying to understand how the trusses are configured where the two roof lines meet at the interior corner.
I'm also looking for a full set of shop drawings for a California Hip Set, I'm seeing a few variations with this hip set I'm trying to determine the most typical way they are put together.
-
For those not familiar with truss hip sets, here is a primer:
6" raised heel with open end jacks and a drop-in purlin frame.
I've also added in the option for open or closed end jacks (sub release "d").
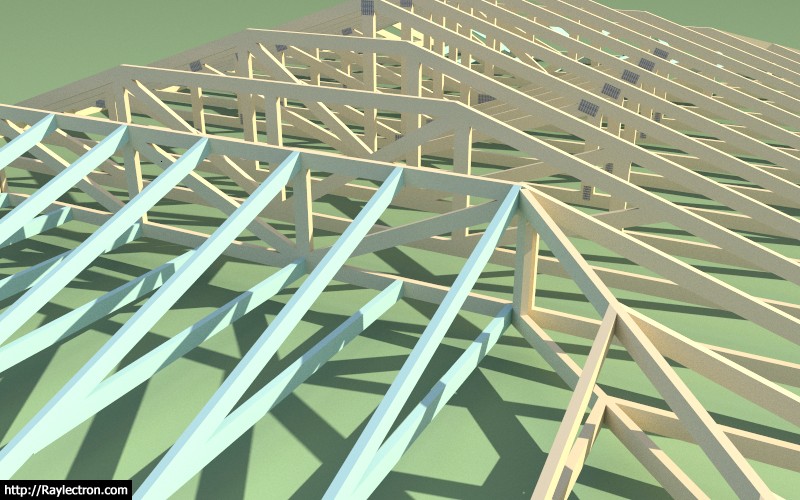
-
Version 1.9.3 - 06.25.2017
- Added Terminal hip set trusses.
- Enabled advanced options for terminal hip sets.
- Added energy/raised heels for terminal hip set (3 variants: wedge, slider and vertical w/ strut).
- Added the option for open vs. closed end jack and single and double ply girders
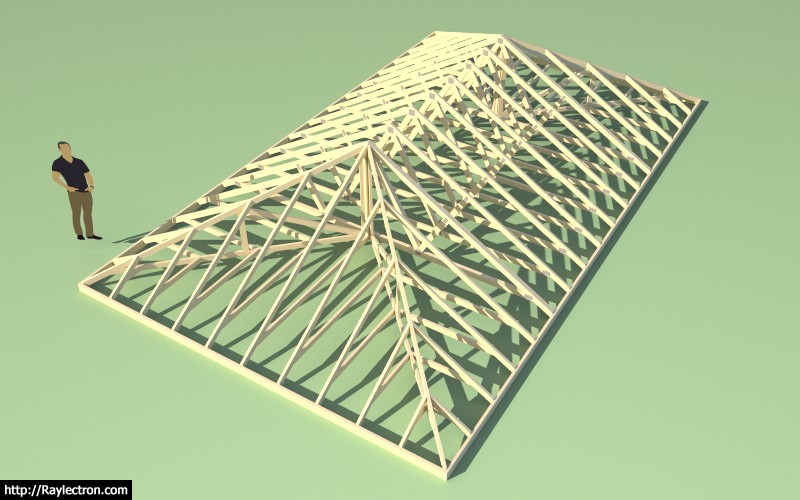
View model here:
3D Warehouse
3D Warehouse is a website of searchable, pre-made 3D models that works seamlessly with SketchUp.
(3dwarehouse.sketchup.com)
This style of hip set is typically used for spans less than 20 ft.
-
Here is a model of the L-shaped hip roof I am in question about:
View model here:
3D Warehouse
3D Warehouse is a website of searchable, pre-made 3D models that works seamlessly with SketchUp.
(3dwarehouse.sketchup.com)
Advertisement








