Using laser distance meters in bright sun
-
@bjornkn said:
Is there a built-in level in the Bosch laser then?
You did not fully understand the point of Gaieus' diagram and comments. As you sweep the meter up and down the distance reading will change. It starts out long reaches its shortest point and then gets long again. The angle where the distance is shortest is a perpendicular to the wall. When the distance is the shortest, you have a 90 degree angle between the wall and the laser line.
-
Also don't forget the 'Similar Triangle' method to get the height of something too tall to measure directly - this uses 'bright-sunlight' because it has to have a shadow - no gadgets required!
-
@roger said:
You did not fully understand the point of Gaieus' diagram and comments. As you sweep the meter up and down the distance reading will change. It starts out long reaches its shortest point and then gets long again. The angle where the distance is shortest is a perpendicular to the wall. When the distance is the shortest, you have a 90 degree angle between the wall and the laser line.
Yes, I understood that. Nice feature
 I was just curious if the Bosch lasers come with a level built-in, as I might be interested in buying one. Could be useful for measuring/calculating a longer reference, for use with tgi3D, than what is easily measured with a 5m tape measure. The longer the reference, the better the precision.
I was just curious if the Bosch lasers come with a level built-in, as I might be interested in buying one. Could be useful for measuring/calculating a longer reference, for use with tgi3D, than what is easily measured with a 5m tape measure. The longer the reference, the better the precision.
My Android phone has a built-in level (but the back side is slightly curved...) and I know that some laser devices are self-levelling, thus I wouldn't be surprised if the Bosch had a level too. And the iPhone probably have an app too?Re. angles and measuring distances:
My question was how to calculate a height if the walls are not exactly vertical, based on only distance measurements from one position.
Gaieus' method will work for vertical walls, and the method with pointing upwards should work too, if there is something there to stop the beam, like a roof, or maybe a board laid on top of a fortress wall etc?
Like TIG explained you need the length of the 2 sides + the angle between them to calculate the third side. As long as the triangle does not have a 90 degree angle (like with a non-vertical wall) you cannot use only distances, but need one angle as well.@Gaieus:
What is the model number of your Bosch? -
@tig said:
Also don't forget the 'Similar Triangle' method to get the height of something too tall to measure directly - this uses 'bright-sunlight' because it has to have a shadow - no gadgets required!
Nice, but it does require a vertical wall and a flat ground, which we rarely have here in Norway

Like this photo of our local fortress shows..
No flat ground, no vertical walls, and no right angles anywhere at all actually. -
There's no sun [therefore no shadows] in Norway for half of the year anyway

How about this make a 'quadrant'
[I know professional 'levels' do it but some duct-tape and bodge never hurt anyone!]
Get an old plastic protractor hold it so the flat edge is at the top and tape a drinking-straw onto the back of it so that it is at 45 degrees and aligned with the central cross-hairs.
Now get a length of string [~50cm] and tape that to the front of it over the cross hairs, hanging down over the curved part. Add a small weight to the end of the string so that it acts like a plumb-bob.
Now if you hold it so that the string aligns with the vertical 90 degree division line on the protractor the drinking-straw 'sight' is at 45 degrees to the horizontal.
You can hold this device but it's much easier to duct-tape it onto a 'staff' about 1.5m long...
You need to walk away from the building to a distance that's an approximation of the height plus a bit.
Place the quadrant on/in the ground with the drinking-straw sight looking at the building and check it's level [string in central on 90 degree marker]. Look through the 'sight' and if you are too near you won't see the top if the building, and you must move away say 1m, if you are too far away you'll see sky and need to move nearer by say 1m. Move back and forth until the required top of the building is dead-center on the 'sight'. Double check for levelness and that the staff is firmly planted. Look along the top [level] edge of the protractor and that will strike the wall in the same horizontal plane. Mark it [an assistant is useful for this but you can set up a ranging-rod against the wall face and estimate up/down along it]. Now run your tape measure from the cross-hairs of the quadrant to the mark on the wall. This gives you the height of the wall above the wall mark. Measure from the wall mark to a fixed level like the floor to give a datum. If the wall is vertical that's itBut if the wall is battered back the true height of the wall above the wall mark is the measured height plus the amount the wall sets back - this is more tricky to establish... [we'll assume an even batter!] you can use the quadrant to estimate this - place the quadrant on the wall base and set it vertical, sight through to a point on the wall and mark it, measure the height of the quadrant above the wall base [h], and the setback of the wall from the center of the vertical quadrant [s]. The ratio is s/h = S/H, where H is the measured-height not actual-height - so the additional height you need to add onto the measured-height is H*s/h...
If the wall is on a hill so that its base will always be above the horizontal plane through your quadrant's first station-point [at the wall's height horizontally away from the wall] then it's more tricky... Instead of a mark on the wall you mark the rising ground and measure horizontally to that, move the quadrant onto that mark and strike a horizontal plane again, if it again hits the ground mark that and measure that and move onto that mark [we are accumulating additional lengths to total up to the total wall height], at some point as we advance towards the wall the mark will be on the wall itself so mark it and measure, now the total distance from adding all of these horizontal distances is the height of the wall above the first station-point - to make adjustments to measure from the wall-base take off the [staff_height * intermediate_stations_number] and add on the distance from the wall-base up to the last wall-mark.Alternatively by some decent surveying equipment

-
@tig said:
Also don't forget the 'Similar Triangle' method to get the height of something too tall to measure directly - this uses 'bright-sunlight' because it has to have a shadow - no gadgets required![attachment=1:1akttagz]<!-- ia1 -->Capture.PNG<!-- ia1 -->[/attachment:1akttagz]
Yeah, that's how a Greek (forget which one) measured the height of the pyramids som 2-2.5 millennia ago. And the pyramids do not have vertical walls either - the point is to take the measurement when the sun is low enough for the pyramid to cast shadow (it will be the point which casts the longest shadow anyway)
@bjornkn said:
I was just curious if the Bosch lasers come with a level built-in, as I might be interested in buying one.
Mine does not. It's a PLR 30 (rather simple one) and it only measures to some 30 metres altogether.
But where it measures from is its "bottom", not from the middle as Roger mounted it (don't know Roger if that affects your accuracy)
[attachment=0:1akttagz]<!-- ia0 -->stick_mount_sm.jpg<!-- ia0 -->[/attachment:1akttagz]

-
Regarding built in levels on laser meters: I believe a couple of the Leica Disto models have on board levels. I have not looked at the whole Bosch line, or Hilti, if either have multiple models.
-
@gaieus said:
Yeah, that's how a Greek (forget which one) measured the height of the pyramids som 2-2.5 millennia ago. And the pyramids do not have vertical walls either - the point is to take the measurement when the sun is low enough for the pyramid to cast shadow (it will be the point which casts the longest shadow anyway)
@unknownuser said:
I don't quite get how he did that using TIGs "similar triangles" method? TIG uses a vertical corner and 2 similar right-angled triangles, where h/H is proportional to d/D. With a pyramid he would have to know/measure the distance to the center of it, no?
@bjornkn said:
I was just curious if the Bosch lasers come with a level built-in, as I might be interested in buying one.
Mine does not. It's a PLR 30 (rather simple one) and it only measures to some 30 metres altogether.
OK. Strange that its specs doesn't mention that shortest/longest feature, while other Bosch lasers in the DLE series does mention it. I have borrowed a cheap "laser" too, but it apparently is only using the laser for pointing, and ultrasonic sound for measuring distance up to 12m

@tig said:
Alternatively by some decent surveying equipment
Good advice

Or, for more fun, speed and lower cost buy a decent plugin/app like PhotoScan
Although I'm a big PhotoScan fan I can see that a laser distance meter can be a very useful accessory too.
Those "laser glasses", is it just a pair of sunglasses with red glass, or does it have to be specifically made for "laser viewing"? -
Well, mine must be much simpler. It definitely does not have a tripod thread. Anywy, for simple, "quick & dirty" measurements (think of GE modelling for instance), mine is also perfect. Or at home when I can place it against a wall to measure the room.
-
Bjorn, regarding the sunglasses or filters, any red will do. However, the closer it is to the red of your laser the better it will work. All you are trying to do is build contrast between your laser dot and the background. The wavelength of most lasers is printed on their safety warning. My distance meter is a 635 nanometer laser. I have a laser pointer labeled between 630-680 nanometer. The distance meter looks slightly more orange than the pointer. However any rich red glass or plastic will work well. A bright sun always implies a rich blue sky. The red glass/plastic will knock down the blue component of your ambient light and continue to pass your red light.
As for tilted walls your argument is incorrect, so I am off to produce an SU diagram to demonstrate.
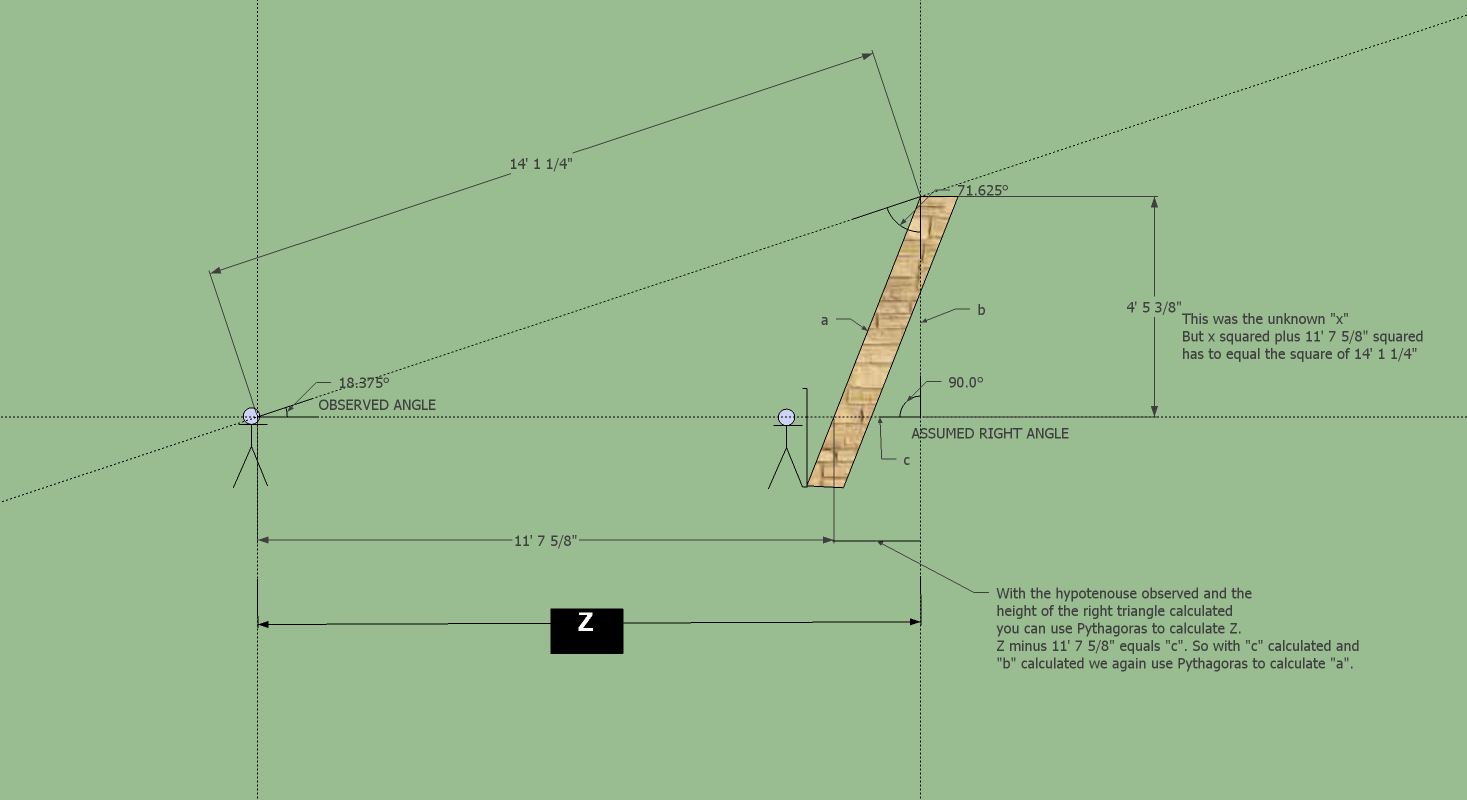
-
@Roger I never said that it couldn't be calculated if you know/measure one angle, the one between the lower and upper measurement.
It is when you don't have any known angle (including the 90 deg vertical wall) the problem arises, because when we don't have a vertical wall (and no angle) we can't know the height because the more the wall slants the lower it will actually be, and still have the same distance - and the smaller the angle gets. -
Slightly related to this topic (barely). I was using "Google" search to locate a store with a cheap angle finder. The map they returned showed Howland Island which was Amelia Earhart's missed refueling stop in the mid Pacific. Now Howland is part of the Phoenix Islands and I live in Phoenix, Arizona so when I am out I will be extra vigilant for anyone resembling miss Earhart.
-
@roger said:
Slightly related to this topic (barely). I was using "Google" search to locate a store with a cheap angle finder.
Slightly related, i rest my Bosch on top of my ~18" angle finder to steady my aim but am attracted to the idea of the stick. Probably bigger than you want, the Denali digital was less than $30 at Amazon a few years back.

-
@roger said:
Slightly related to this topic (barely). I was using "Google" search to locate a store with a cheap angle finder.
I suppose this implies that the iOS theodolite app didn't work very well then?
-
@bjornkn said:
@roger said:
Slightly related to this topic (barely). I was using "Google" search to locate a store with a cheap angle finder.
I suppose this implies that the iOS theodolite app didn't work very well then?
Not at all. I let the project vaporize because I didn't want to head out to the middle of the desert in mid summer. No mad dogs and Englishmen out in the midday sun for me (at least not in Arizona). Should I get my hands on a proper cell phone that can run the app you can be rest assured that I am eager to try it.
However I am even more eager to try out the TFI3D (SP?) product.
-
I thought you had an iPhone to test it on?
I did a little test with a similar Android app called GeoCam. It does show pitch/azimuth as well as roll, but it uses no decimals, just integers
@unknownuser said:
However I am even more eager to try out the TFI3D (SP?) product.
I suppose that would be Tgi3D? Or could it be something else?
-
I just found a suit of tools on Android Market: Smart tools toolbox:
https://market.android.com/details?id=kr.aboy.tools&feature=related_apps
It can measure distance, height, width, slopes/angles, compass (as well as dB and vibration!) etc..
Looks interesting, although I doubt that the precision can be very high..
Guess it's time for my first purchase on that market... -
hey, that looks like a useful compact stick, need to put in my shopping cart.
I have had the DLR165 for a few years, you choose from four locations where the measurement will originate.GLR225 comes with a bubble.

edit: photo switch to actual

-
I've been reading this post with interest as I have a Disto and have been looking for a way to add horizontal measurement, but most systems are very buggy and/or very expensive, but some Swiss cavers have added a third-axis upgrade to a Disto A3 http://paperless.bheeb.ch/. Apparently it will work on other lasers, but the Disto has greater accuracy and functionailty (and the Disto A3 has enough room within the case for the upgrade). The device uses the Disto's memory to hold the resulting point cloud prior to Bluetooth transmission to PDA for field-drawing or for building into a drawing later.
.I'm considering getting someone to build me one of these, as it's essentially a poor-man's electronic theodolite, and would revolutionise field work. I understand they cost around 150 Euros to build (and the site has full specifications and construction info for the electronically-minded)
Just thought you might be interested in this.
Advertisement







